In the realm of constructing sophisticated physical models, particularly those grounded in gravitational theories, a commonly employed technique is the gluing of two distinct spacetimes along a shared interface. This approach achieves the desired topological and geometric configurations, offering a powerful framework for exploring complex physical phenomena. A quintessential example of this methodology is the braneworld model, which postulates the existence of a brane — a codimension-1 timelike hypersurface embedded within a higher-dimensional spacetime known as the bulk. The brane, often referred to as a thin shell, serves as an elegant explanation for several profound physical conundrums, including the Hierarchy problem, which underscores the puzzling disparity between the strength of gravity and the other three fundamental forces of nature. The matter present on the brane generates an energy-momentum tensor that is proportional to a Dirac delta function within the bulk, leading to discontinuities in key physical quantities across the brane, such as its extrinsic curvature. This bulk/brane structure, therefore, can be interpreted as two distinct spacetimes seamlessly joined along the brane. However, to maintain consistency within the gravitational field at the junction, specific matching conditions must be rigorously satisfied. These conditions, first systematically formulated by Darmois and Israel in the context of classical general relativity, have since been extended to accommodate a variety of modified gravity theories. Nevertheless, all such extensions have traditionally been confined to configurations involving the junction of only two bulk spacetimes.
At the heart of contemporary physics lies the quest to quantize the gravitational field, with the ultimate aim of formulating a comprehensive theory of quantum gravity. While this monumental goal remains on the distant horizon, certain perplexing questions provide glimpses into the underlying nature of quantum gravity. Chief among these is the celebrated black hole information paradox. In the 1970s, the eminent physicist Stephen Hawking predicted that black holes gradually evaporate through the process now known as Hawking radiation. According to the no-hair theorem, Hawking radiation depends solely on three fundamental parameters of the black hole — mass, charge, and angular momentum — each of which is highly degenerate, implying that the radiation is in a mixed state devoid of any information. However, if a black hole begins its evolution in a pure state containing information, this suggests an apparent loss of information over time, giving rise to the paradox. According to the unitarity of quantum mechanics and Liouville's theorem, a pure state can only evolve into another pure state, and therefore, information must be conserved. This discrepancy lies at the core of the black hole information paradox. With the advent and maturation of the holographic principle, it has become widely accepted that information is indeed preserved. In recent years, researchers working within the framework of 2D Jackiw-Teitelboim (JT) gravity have demonstrated how information-preserving Hawking radiation can arise through contributions from a multi-boundary spacetime configuration, commonly referred to as replica wormholes, in the gravitational path integral. However, the configuration arising from the junction of multiple spacetimes along a common interface forms a multi-boundary structure that is topologically distinct from replica wormholes and has yet to be fully considered — despite its potential significance in the gravitational path integral, which involves summing over all possible topologies.
For the first time, groundbreaking research spearheaded by Professor Li-Xin Li from the Kavli Institute for Astronomy and Astrophysics at Peking University, alongside his Ph.D. student Jia-Yin Shen, and Associate Professor Cheng Peng from the Kavli Institute for Theoretical Sciences at the University of Chinese Academy of Sciences, delves into the dynamics of multi-boundary spacetimes formed by gluing multiple bulk spacetimes along a shared interface. Their innovative work has been featured in the famous journal Physical Review Letters.
One of the primary challenges of this research was to derive the junction conditions at the interface where multiple bulk spacetimes converge, termed multiway junction conditions, which serves to distinguish the classical Darmois-Israel conditions. In contrast to the classical case of two bulks, the junction of three or more bulks results in the absence of a differentiable structure at the interface — a critical element for defining various physical quantities. As a result, the Einstein tensor, which typically describes the gravitational field, cannot be defined at this interface. To overcome this obstacle, the researchers developed a novel formalism known as "booklet geometry," utilizing a key methodological innovation termed the reverse extension method. In this framework, each joined bulk spacetime is referred to as a "page," while the entire composite structure is labeled a "booklet."
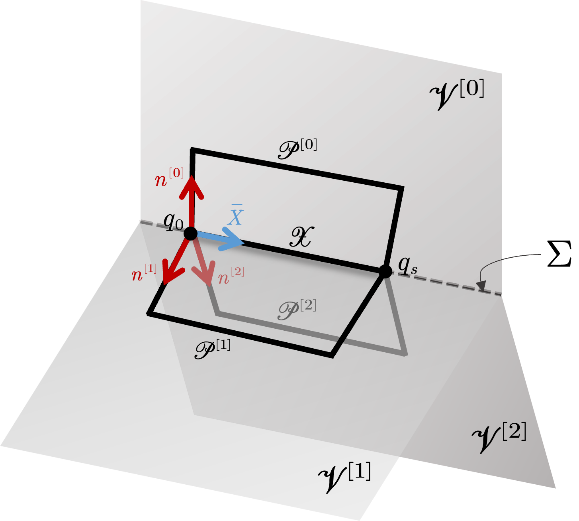
Figture 1: Structure showcasing the gluing of three bulk spacetimes
Visualized in Figure 1 for the case of three pages, the booklet is embedded into an external manifold of sufficient dimensionality, enabling the recovery of a differentiable structure. Through meticulous analysis, it was uniquely determined that the dimension of the external manifold is equal to the dimension of the interface plus the number of bulks, minus one. At this juncture, a linear combination of normal vectors to the interface in all pages exists, although this combination may degenerate at certain singular points. These singularities, however, can be disregarded as the junction conditions are local and can be determined within an open set. By arbitrarily selecting one page — referred to as the "gauge" —and extending it in the reverse direction of its normal vector, an auxiliary bulk is constructed. The geometry of the external manifold is then determined by all the pages except the gauge. Through constraints and reductions, the auxiliary bulk's geometry is fully determined. Finally, by applying the classical Darmois-Israel junction conditions between the gauge and its reverse extension, the definition of the Einstein tensor applicable to the entire booklet is circumvented, but the multiway junction conditions across all pages are obtained, a process illustrated in Figures 2 and 3.
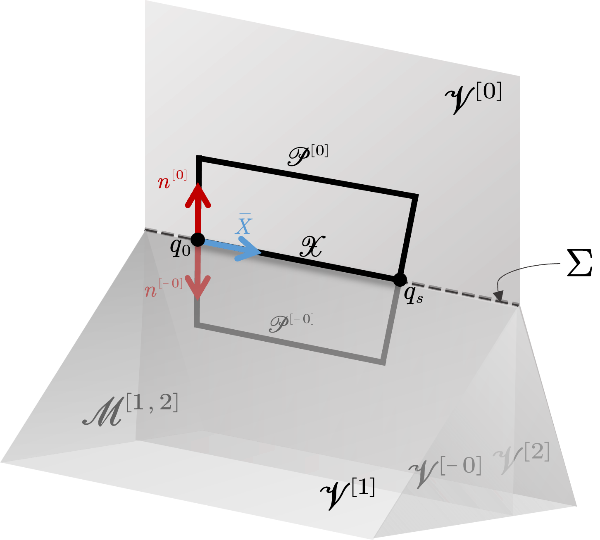
Figture 2: Embed the booklet, comprising three pages, within an external manifold, and then arbitrarily choose one page for reverse extension.
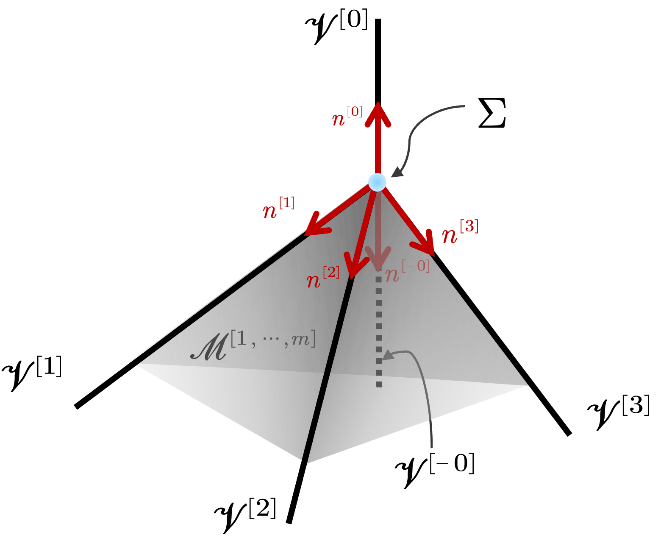
Figture 3: Apply the reverse extension method to a booklet with any number of pages.
A fundamental question arises from this methodology: does the arbitrary selection of the gauge affect the resultant multiway junction conditions? If different choices of gauge lead to differing junction conditions, this would imply that these conditions are not reflective of a true physical law. Therefore, the multiway junction conditions must remain invariant under any choice of gauge, thereby fully determining the form of the linear combination of normal vectors: the sum of all normal vectors must equal zero. This linear combination is referred to as the continuity condition of the normal vectors, a dual counterpart to the multiway junction conditions. While the latter governs the gravitational consistency of the bulk spacetimes and is observable by those within the booklet, the continuity condition restricts the embedding of the booklet within the external manifold, remaining imperceptible to observers within the booklet itself.
Moreover, the researchers independently derived the multiway junction conditions through the application of the variational principle. By considering the action of each page as the Hilbert-Einstein action, supplemented with the Gibbons-Hawking-York boundary term, and assuming the total action of the booklet is the sum of the individual pages' actions, the principle of least action was employed. Retaining only the boundary terms, the researchers obtained the same multiway junction conditions, thus cross-verifying their findings. Finally, the scope of their work extends beyond classical gravitational theory. The multiway junction conditions were generalized to dilaton gravity models and JT gravity models, which are pivotal in the ongoing exploration of the black hole information paradox. These extensions have yielded a cascade of critical insights.
--------------------------------------------------------------------------------
This work was supported by the National Natural Science Foundation and the Fundamental Research Funds for the Central Universities.
Original Paper:
Shen, Jia-Yin, Cheng Peng, and Li-Xin Li. "Multiway Junction Conditions for Spacetimes with Multiple Boundaries." Physical Review Letters 133.13 (2024): 131601.
https://journals.aps.org/prl/pdf/10.1103/PhysRevLett.133.131601
Shen, Jia-Yin, Cheng Peng, and Li-Xin Li. "Multiway junction conditions: Booklets and webs." Physical Review D 110.6 (2024): 066021.
https://journals.aps.org/prd/abstract/10.1103/PhysRevD.110.066021